Filling Polytopes
Recent changes
23 Mar 2023. A seductive fallacy added.
14 May 2021. Completed. Includes a major rewrite with various corrections.
Abstract
In the theory of polygons, polyhedra and polytopes in general, the filling (or otherwise) of their interior is of fundamental importance. Traditional theory ignores filling, and so is incomplete. Moreover the abstract and topological approaches do not align. These issues are resolved by treating a polytope as a bounded topological manifold in its own right.
Contents
- Introduction
- A seductive fallacy
- Problems with traditional theory
- Problems with holes
- Inside and outside
- Polygons as surfaces
- References
Introduction
The basic idea of polygons and polyhedra may be generalised in other sets of dimensions as polytopes. A polygon is a polytope in two dimensions, or 2-tope, and a polyhedron is a 3-tope. The reader who is only familiar with the usual three dimensions should be able to understand what follows, while students of higher dimensionalities should also find it relevant. In higher dimensions, a 4-tope is a polycell or polychoron, and so on. In lower dimensions, a 1-tope is a closed line segment or ditelon, and a 0-tope is a point or monon.
Traditionally, we tend to say that a polytope of a given dimension is built up from polytopes of the next lower dimension. For example a polyhedron is built up from polygons, which form its faces.
The filling of polytopes has not been thought to have much significance. Different writers made different assumptions (if any), or adopted different graphic conventions, as to whether their polytopes were solid or empty. It seldom seemed to matter much at the time. But when one looks carefully, one finds a fundamental muddle to be sorted out – so fundamental in fact, that I now believe that the proper description of any polytope must include its filling.
A seductive fallacy
There is a seductive narrative on the relationship between the inside and outside of a polyhedron. Consider the basic case of a convex polygon. We often treat its interior as a set of points. Such a point may be identified by drawing a ray from the point to the polygonal boundary. We then rotate the ray about the point, such that its point of intersection with the boundary travels along it until, after a full turn, the point of intersection has completed a full circuit back to its starting position. Thus, the interior may be defined as the set of points about which the polygon winds.
More rarely, we treat the exterior plane as the set of lines which do not meet the polygon. When we polarise the polygon in a concentric circle, we find that the interior points polarise to the exterior lines of the dual polygon and the exterior lines to the interior points of the dual. (As an exercise, what is the polar equivalent of constructing and rotating a ray in this manner? Why do you end up with a point traversing a line twice? Hint: just as the point of intersection traces all the edge segments, so, dually, the line of joining must traverse all the vertex angles.)
The idea readily extends to polyhedra. Here, we treat the exterior space as a set of planes, and these turn out to be reciprocal to the interior points of the dual polyhedron. And so on in higher dimensions.
It is often said that this idea can be easily adapted to self-intersecting figures such as stars. Poinsot argued that the regular stars are convex because all their vertices are convex, and Cayley developed the idea of interior density, according to how many times the boundary winds or wraps around any given interior point. But for non-orientable polyhedra, one cannot establish anything more than a parity value for the density; Cayley's idea only works for orientable figures. As far as the reciprocity of inside and outside goes, the situation is even worse. For any star, indeed for any non-convex figure, we find that certain interior points reciprocate to lines which do meet the dual figure. The convex narrative may be seductive, but in the general case it is flawed and unworkable.
Problems with traditional theory
So why does it make a difference whether, say, a polygon is filled or hollow? A polygon unambiguously divides the Euclidean plane into inside (smaller) and outside (larger) regions, which is enough for most people. Let us use some polygons to construct a polyhedron. Six solid or filled squares make a closed cube, or six stick or hollow squares make a skeletal cube. Both of these cubes have very respectable credentials. We can mix open and closed squares to make an open box, using say five filled squares plus one hollow square. This is a little messy int heoretical terms, but all six sides are perfectly respectable polygons, so why not? Fans of filled polygons would say that the open end is not a side so the open box is not a polyhedron, but are they right? Fans of skeletal polygons would say that the fillings are an irrelevant afterthought so the open box is a cube and just as much a polyhedron as its skeletal sibling, but are they right? To get messier, are these six sides all the same kind of polygon or not? They are all squares having the same structural arrangement of edges and vertices, and so traditionally all have the same mathematical definition. But one square is obviously different in some way for the box to be open on that side. Topologists say that the various cubes described are not homeomorphs; this is no simple sophistry on my part. We are forced to conclude that traditional definitions of a polygon are incomplete; in describing these squares fully we must also consider whether they are filled or not.
How has this shortcoming in polytope theory been missed for so long? I believe that it comes down to the way we visualise polytopes. Figure 1(a) shows a star pentagon, and 1(b) a decagon having the same external form. A two-dimensional flatlander standing next to them would not be able to see inside them and tell them apart, and might well consider the distinction trivial. Yet to us three-dimensional mathematicians the difference is obvious. 1(c) shows a star polyhedron. Is it constructed from 12 star pentagons like 1(a), 12 nonconvex decagons like 1(b) or perhaps 60 isosceles triangles? Now it is us who cannot see inside to tell the difference, yet to a four-dimensional demon it would be obvious.
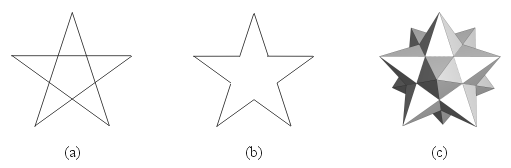
Figure 1 : Star pentagon, nonconvex decagon and small stellated dodecahedron
A prison makes a nice analogy. Traditionally, a prisoner is held captive whether they are inside a building or in the open air such as in a walled compound; whether the area is roofed is not significant, they cannot climb over the walls. Consider a prison built to the plan of Figure 7(b), having six cell blocks around a central compound: no prisoner can escape from this compound. But now suppose that a friendly helicopter flies over and lowers a ladder – a prisoner in the compound can climb into the third dimension and escape, while a prisoner in their cell can not.
Twentieth-century theorists were much concerned with the combinatorics of point sets, with sorting and ordering the points in appropriate ways. They saw the cube as its set of eight corner points or vertices. An edge was just a point pair, a face a cyclic set of such pairs. Figure 2 shows three ways to fill a cube. In 2(a), just the 0-topes (vertices), have been filled in. The diagram is not very instructive, in fact it might even be showing a stella octangula (a regular compound of two tetrahedra) or any other figure having the same vertices. 2(b) and 2(c) are much more understandable, and both are commonly used.

Figure 2 : Three different fillings of the cube
Grünbaum was aware of such issues [1]. His main concern at the time was with such point sets. However, like many authors on the subject of vertex sets, he avoided vertex-only diagrams in the style of 2(a), preferring to depict the point pairs as edges after 2(b). He explicitly ignored fillings such as 2(c).
For the drawings to adhere strictly to the theory, one should also ignore fillings such as 2(b). But of course they would then have been next to useless to Grünbaum's readers. In particular, since he was dealing with non-convex polyhedra he did not want anybody to be caught in such confusions as whether 2(a) was a cube or a stella octangula. Our four-dimensional demon would most likely level the same criticisms at 2(b), finding the faces of 2(c) far more intelligible. (To be fair to the combinatorialists, they were seeking to isolate the structural essence of a polyhedron regardless of appearance, a line of study which has culminated in the modern theory of abstract polytopes.)
On the other hand, when Coxeter et. al. analysed the stellations of the icosahedron [2], they treated the resulting forms as solid lumps. As a result they recognised neither internal structure, such as that seen in Figure 1(a), nor the falseness of certain vertices and edges where cells touched, again seen at the crossing points in 1(a). Because of this their selection of 57 such icosahedra, though employing some innovative mathematics, is otherwise well-nigh meaningless [3].
There is already a problem with 2(c) in that we cannot tell whether it is an empty box or a solid. Traditionally we have not worried about this. But now look at Figure 3, which shows three fillings of a tesseract or hypercube, a 4-tope constructed from eight cubes. Figure 3(a) shows only the vertices and is almost useless. Figure 3(b) shows the edges and is much better; our eyes certainly need those edges filling in, don't they! Here we can immediately see the general structure, and with a little study we can discern the eight individual cubes, distorted to varying degrees by the projection from 4-space to-2 space. Figure 3(c) is now less helpful than before. Because it is a convex polytope, our four-dimensional demon would be able to see the full structure quite clearly, and whether each individual cube was solid, but if they were then it would not know whether the interior hyperspace was filled or not. However not only can we not tell whether the cubes are filled or if the 4-tope itself is solid, but even if we could walk round a 3D model we would find that the outer faces have obscured much of the polytope's structure from view. So students of the higher dimensional polytopes often stick to edge-fillings only.
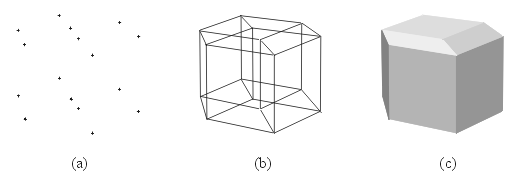
Figure 3 : Three different fillings of the tesseract
A feature of computer simulations reinforces this habit. Computers are very useful for manipulating higher-dimensional polytopes, because they can be easily programmed to cope with the extra dimensions. When it comes to displaying the polytope, it is relatively easy to program the drawing of lines between the vertices, but much harder to program where solid faces are visible and where they obscure each other, never mind finding false edges where faces of a nonconvex polytope intersect. So we have tended to stick with what is both easiest to program and clearest to look at, and just draw the edges.
So you see, which n-topes we traditionally fill in depends very much on how we like to visualise them. Our eyes need filling of ditela most of all, fillings of polygons and polyhedra come and go according to our line of study, but above that we very rarely fill things in. This filling of polytopes according to the way our senses work is not mathematically rigorous. In fact, it's amazing that theoreticians have gone along with it for so long. We need something better.
Problems with holes
Here's a more subtle problem. Traditional polygon theory does not concern itself with certain kinds of hole. Regions surrounded by the bounding edges are defined as being in the interior, there is no distinction as to whether a region is filled or not. As a result the theory is not able to describe certain kinds of polyhedral structure, such as the hole in Figure 4(b).
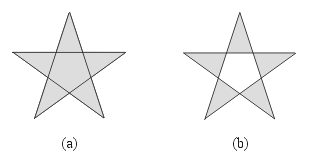
Figure 4 : Fillings of a star pentagon
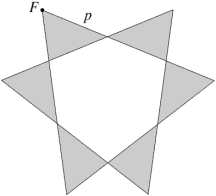
Consider the two star pentagons in Figure 4. Figure 4(a) shows the filling usually given by mathematicians, which distinguishes interior and exterior regions. The central region is said to have a density of 2 because the boundary winds round it twice. Seems reasonable? Well, many general-purpose graphical drawing programs for computers will give you Figure 4(b). Programmers seem drawn to the logic that a boundary is a division between inside and outside, no matter where it occurs. This time, mathematicians and programmers take opposing views.
It is possible to find polygons naturally having the form of Figure 4(b). Figure 5 shows two views of a polyhedron with pentagonal pyramid symmetry, having five kite faces and five cross-quadrilaterals. Although this example is rather flat, the general shape resembles a hollow horn, so we may call it a keratinoid or cornoid. The vertex figure of the central apex is a pentagram whose centre lies outside the polyhedron, and so is hollow.
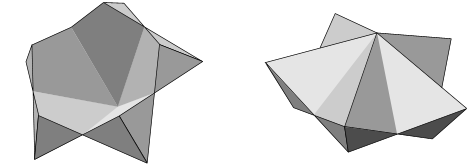
Figure 5 : Pentagrammatic keratinoid
Figure 6 shows two equivalent views of the dual polyhedron, the star pentagonal cuploid. This polyhedron is usually depicted with a filled-in pentagram, as here. The central region is a membrane separating the inside and outside of the "cup". This is usually justified on the grounds that the region has denisity 2 and so acts as a kind of double-skin. This led Cayley to realise that the core of the small stellated dodecahedron has density 3: once for the surface of the outer spike, and twice for the centre of the pentagram surrounding the core [4]. The densities of surfaces and of the regions of space beneath them cannot be treated separately. Returning to the cuploid, notice that the regions of space on either side of the membrane both have density 0. This must mean that the membrane also has density zero, otherwise the densities either side would be different, after the manner of Cayley.
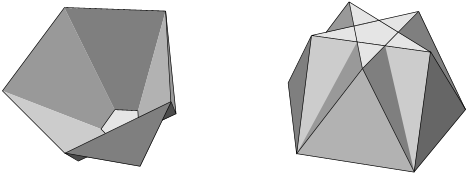
Figure 6 : Pentagrammatic cuploid
In order to understand how this can happen, we need to take into account the fact that the polyhedral surface is non-orientable: that is to say, we cannot separate out an "inner" side from an "outer" one. Try it – start on one side, say the outside, and by tracing up over the rim and down again it is possible to end up on the inside (this is because the surface twists in a particular way; topologically we observe that it incorporates a one-sided Möebius strip). In these circumstances, it is not possible to assign densities to deep regions of the interior in the way that Cayley did for the regular star polyhedra. The best we can do is to assign a parity (0 or 1 modulo 2) to each region. Grünbaum sent me the following explanation:
Let P be a polygon in the plane. At this point let's think of it as a bunch of segments. If you insist on "sides" being just pairs of points, one needs to adjust the language, but not the idea. Let L be the union of the lines determined by the sides of P (disregard the ones of zero length). The complement of L in the plane is a finite family of open regions R1, R2, ... , Rk. Take a point V in one of these, and a ray H that issues from V and does not go through any vertex of P (thus only finitely many rays are disallowed), and count how many sides (edges) of P are crossed by H. The parity of that number determines whether d(V;H) , the density at V with respect to H, is 0 or 1. Next, observe that d(V,H) does not depend on H, by considering what happens when you rotate H about V: Most of the time the same sided are crossed, but when H passes a vertex some or all sides can be changed and their number as well -- but all leaves the parity (that is, d(V,H) ) unchanged. Call that value d(V). Next, observe that d(V) does not depend on V as long as we stay in the same region Rj, since each of these region is convex and we can take the ray that starts at one point and goes through the other to see that d(V) is the same for them. Finally, let Ri and Rj be regions that have a common segment in their boundaries. Consider two points, one in each region, such that the segment connecting them intersects the segment separating them. When proceeding along a ray from one of the points to the other, the parity of d(V) will stay the same or change according to the parity of the number of edges that have to be crossed (the segment may belong to several edges of the polygon).
Now that we know what happens in the plane, we can go to polyhedra. Consider the complement of the union of planes that contain the faces, and for any point V in the complement determine d(V) as before, but for each point at which the ray crosses the plane of a face, observe what is the parity of that point w.r.t. the face. Since any two rays starting at the same point are in a plane, the intersection of that plane with the polyhedron reverts us to the earlier situation on noticing that d(V) is the same whether calculated as stated in the preceding sentence, or in the preceding paragraph. And that's all. Clearly, one can go up in dimensions. Also, clearly, in some cases it may be possible to give sharper counts than just the parity -- this certainly happens with orientable polyhedra.
This is pretty much how the programmers do it. Some even go so far as to offer the two options for counting density; choosing simple parity leaves the figure open, choosing a nonzero absolute count fills it in. Luckily for them, polygons are always orientable, so Cayley's approach will work for them as well as Grünbaum's does.
However densities higher than 1 are only an option ayway, and cannot be taken for granted. Unlike polygons and orientable polyhedra, the option to apply them to the cuploid is not even available.
Here is another polyhedral example. Figure 7 shows stellation f1 of the icosahedron [5]. Each face is bounded by two overlapping regular triangles. The equivalent polyhedron having fully filled faces is the well-known regular compound of ten tetrahedra, but here the central region is empty. By failing to fill this central region of each two-dimensional face, the topology of the three-dimensional figure is drastically changed and it becomes a toroid of genus 11.
 |
 |
|
| (a) | (b) | |
Figure 7 : Stellation f1 of the icosahedron and its face diagram |
||
The two regular triangles bounding each face have six vertices in all. The central hole has no vertices, and as such cannot be described by traditional theory. Adding vertices at each crossing point would destroy the elegant simplicity and symmetry of the faces, and would also result in a very different structure.
Having seen that our partly-filled pentagram and triangle compound are theoretically sound, how are we to describe them, so as to differentiate them from the fully-filled ones? Considering the pentagram, we cannot add more vertices around the pentagonal central hole, for this would drastically change the figure – making it a set of five vertex-connected triangles, which is not what we meant. If we say that we now have six polygons – five triangles and a pentagon – then we have the kind of mix 'n' match filling we met earlier with the open box. If we say that the hole has no vertices, then conventional theory has no way to describe it, though computer programmers evidently have. If you don't believe me, make a star pentagon from five crossed sticks joined only at their ends. Now glue on five paper triangles, leaving the central hole empty. Have you changed the sticks? No, they are the same star pentagon as before. Using any one conventional definition of a polygon, can you give a consistent description of the figure in your hand? It seems not.
We can now understand that when a two-dimensional polygon (or set of overlapping polygons, as in this example) is used as the face of a three-dimensional polyhedron, its filling has a fundamental significance. Traditional theory cannot describe the difference between the faces, and so cannot account for the differing topologies: because it ignores fillings and holes, it is simply not up to the task of describing such polyhedra.
Inside and outside
Any ditelon includes its two bounding vertices. Two ditela may share a common vertex, so mathematically we can order three or more to form a closed circuit, or polygon. Does such a polygon have an inside and an outside? Not necessarily – if the 2-space it inhabits is the surface of say a toroid, it might pass round the toroid as in Figure 11. In this case there can be no filling. The same phenomenon technically happens in the projective plane, though it is less obvious. (You might think this a bit of an irrelevance, but the theory of such closed cycles is of fundamental importance to topology – and hence also to non-convex polytopes – and is known as homology.)
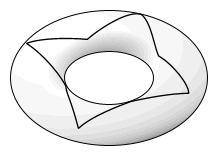
Figure 11 : A star octagon drawn on a toroid.
But usually (i.e. in the Euclidean plane) we obtain the familiar dividing of 2-space into smaller (inside) and larger (outside) regions. We have seen several ways to fill a star polygon. Here they are again in Figure 12, along with a few more. 12(a) gives the data needed to calculate areas for the rest.
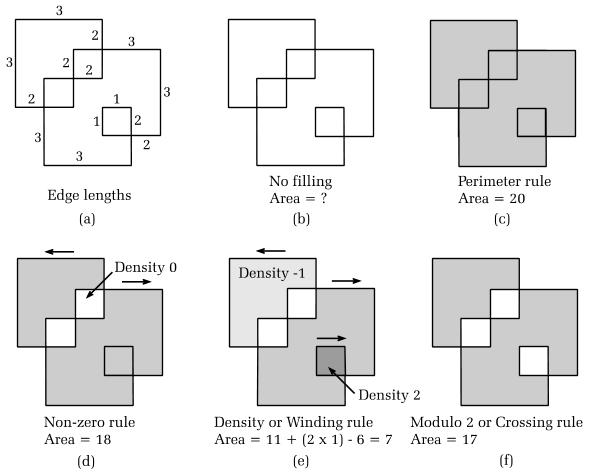
Figure 12 : Different rules produce different fillings
The 14-sided polygon shown was chosen to higlight the way that different fillings affect the calaculation of the polygon's area. Mathematicians disagree as to how the area of a polygon should be defined, which comes down to disagreeing about how to fill polygons. One common method for finding areas of non-convex polygons divides the figure into triangles. Mark any point. Draw a line connecting each vertex to the point). It then adds or subtracts the area of each triangle, according to which way round the triangle winds (Choose a direction of traversal for the polygon, and mark each side with an arrow as you traverse it. Traverse each triangle in the direction given by the connected arrow). This yields a double-count of area for the region of density 2, essentially Cayley's method and the same as the winding rule 12(e). Well, that sounds reasonable – after all it is covered twice. Johnson's abstract theory defines the interior, or body, as the set of points which the perimeter contains, as 12(c) [6]. This yields a single count for any region, regardless of density. The computer algorithm described by Grünbaum yields 12(f), while his point sets hit the buffers with 12(b). Oops! Who is right?
Polygons as surfaces
Notice that I have been talking as if there is a single "polygon" that can have different "fillings." It seems reasonable to treat all the objects in Figure 12 as essentially the same polygon rather than different ones. Otherwise, would we not have great difficulty in defining what a "polygon" is? And having said that, is it reasonable to say that we may fill our polygon in any way we choose, and that different fillings suit different purposes? For example the open cubic box we met earlier has six identifable squares, so why not just say that even though five are filled and one is not, they are all the same polygon?
But consider that open face as a different colouring – suppose we have a blue box with a red lid, and we put the lid back on. Now, if we rotate the cube a quarter-turn so that it lies on its side, the coloured face is no longer in its original position and the mathematical symmetry of the uncoloured cube is brloken. Such colourings play an important part in polyhedral theory, and we recognise different colour arrangements as having different symmetries. For example our coloured cube is no longer a regular polyhedron because it does not have a regular symmetry. This argument only makes sense if we treat the red and blue squares as different polygons.
We are driven to a view in which many different polygons may have the same shape but different fillings. We can say that the circuit of edges is the boundary, and the filling the body, of some surface region. We might imagine cutting that shape out of a sheet of very stretchy rubber, twisting it up and then squashing it back down into the plane again.
Figure 13 shows how a solid disc may be twisted up and flattened down to form a star pentagon after Cayley polygon. When the sheet is twisted round and flattened, it forms a double-circuit - and angle of 4π or 720° - around some point in the otherwise smooth geometry of the surface. To preserve symmetry this double-wound point is pushed to the centre of the pentagon, shown as P in the diagram.
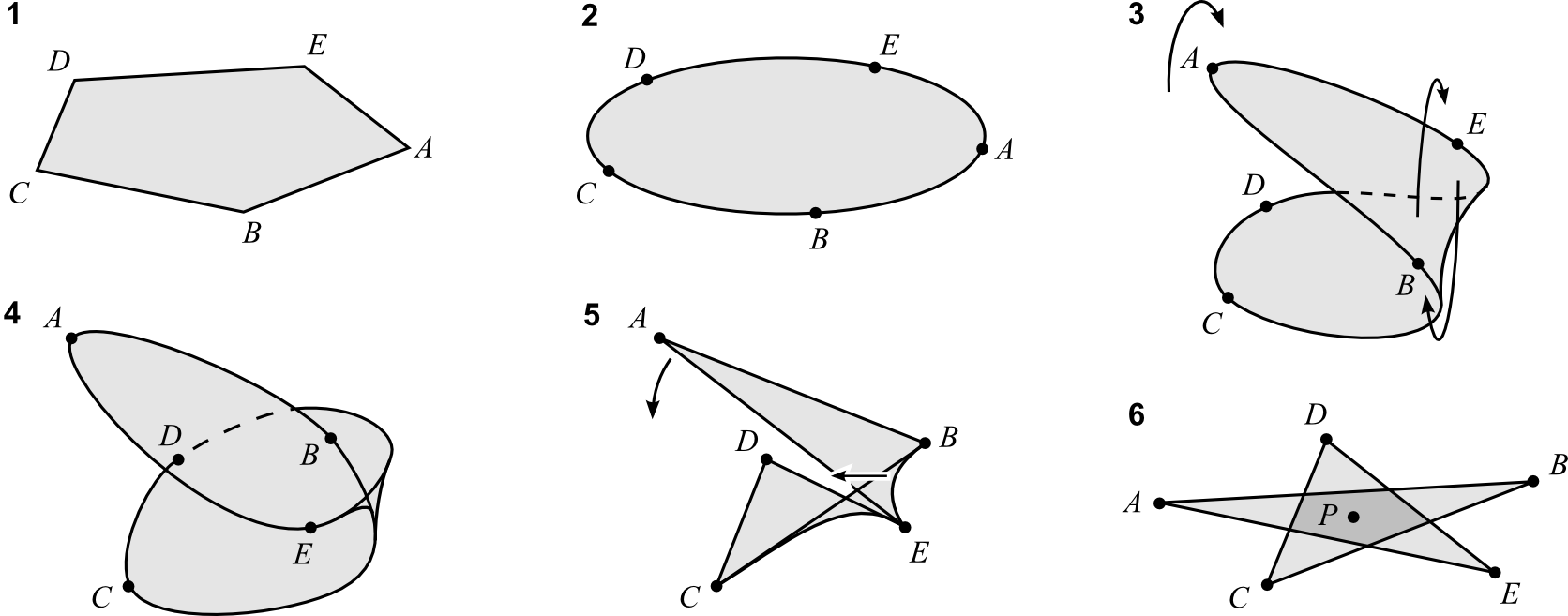
Figure 13 : Morphing a disc-like pentagon into a Cayley star
What if we begin with a a Möbius band instead of a disc? Figure 14 shows how it can also be bounded by a polygonal circuit and squashed down into a star polygon. However this time it has a hole through the middle. This matches the polygon seen in the vertex figure of the cornoid and completes its definition.
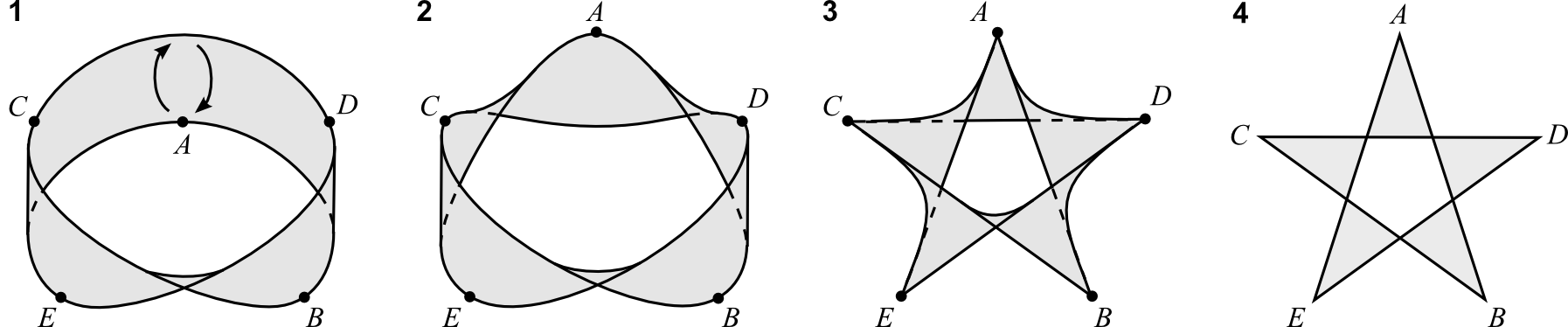
Figure 14 : Morphing a Möbius band into a hollow star
Topology is again on top of the idea. Indeed, the biggest surprise for me is that nobody has, as it were, joined the dots between the theories before now. Topology calls such a region a bounded manifold and the material it is cut from "magic rubber". Note that such a piece need not be flat like a disc but may be say a twisted Möbius band. A polyhedron is similarly a solid block bounded by its surface and also pliable enough to be twisted around. Thus, topology offers a rigorous mathematical link between the purely abstract structure and the geometric appearance. We can say that a given polytope is defined not only by its structure but also by the topology of its body. At last! A complete and rigorous definition of polygons, polyhedra and all the rest!. I call this missing link morphic theory and the half-real topological figures it concerns itself with are Morphic polytopes [7].
References
- B. Grünbaum; Polyhedra with hollow faces, Proc of NATO-ASI Conference on Polytopes ... (Toronto 1993), ed T. Bisztriczky et al, Kluwer Academic (1994) pp. 43-70.
- H. S. M. Coxeter, et al; The fifty-nine icosahedra, 3rd Edition, Tarquin (1999).
- G. Inchbald; In search of the lost icosahedra, The Mathematical Gazette 86, July 2002, p.p. 208-215.
- A. Cayley; "On Poinsot's Four New Regular Solids", Philosophical Magazine, 17 (1859) pp. 123-128.
- G. Inchbald; Towards stellating the icosahedron and faceting the dodecahedron, Symmetry: Culture and Science Vol. 11, 1-4 (2000) pp. 269-291.
- N. Johnson (ed. G. Inchbald); Polytopes – Abstract and Real, web page (2008).
- G. Inchbald; Morphic Polytopes, web page. Subject to revision.